It takes time to develop the equipment skills we are looking for in this context. This lab builds on and exercises the techniques from the RC labs in previous weeks.
Purpose:
To explore the relationship between what we calculate/analyze in an academic setting and what that looks like in the wild where the resistors and capacitors are not some Purdue like object but rather free range devices with a mind of their own.
Now lets consider the behavior of the inductive equivalent of the RC circuit we looked at last week. Because typical inductances tend to be quite small I put a 100 ohm resistor in the circuit to limit the current flow so the function generator won't freak out. (you should calculate the maximum current flow under these circumstances and the probable voltage from across the 1 ohm resistor so you know what to expect for oscilloscope scales). Given the inductors we have available we will be unlikely to be able to generate the full frequency dependent behavior that we did last week so be prepared to measure smaller phase shifts.
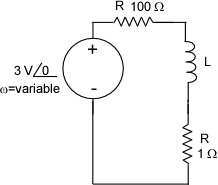
Procedure:
1) Wire the illustrated circuit on a small project board with what ever Inductor I have for you. Because I will be asking you to swap different inductors into the circuit use your clip leads to connect the inductor. We will use the function generator as the power supply. Be careful to define a clear ground point in your circuit layout as well as where you will connect the function generator. After having the circuit verified by the instructor you may set up the function generator and begin your measurements.
You will be making measurements of the input waveform and the output waveform across the 1 ohm resistor. If you can do these both simultaneously it will make things go quicker. To accomplish this you will need to use both "channels" or inputs on your oscilloscope simultaneously. There are some additional settings you will need to check and set to make this happen. Check with your lab instructor if you have questions.
2) Set up your function generator to provide a 3 V peak to peak sine function at an w of 10 kHz (just for starters). Use the oscilloscope to verify this set up. Calculate the expected AC voltage and phase shift, as a function of inductance across the 1 ohm resistor for your circuit.
3) Measure and observe the output voltage across the "load" resistor and determine the phase shift between the source and the load. Is the current leading or lagging and how do you know? You should have a clear expectation for both the amplitude and the phase shift of the load voltage from the previous step. Sketch the traces you observe on the scope and indicate the amplitudes and phase relationships.
4) For low frequencies (10 kHz or less) what is the phase and amplitude relationship?
5) Find a frequency at which you can measure the phase shift and verify that the phase shift is appropriate to your inductor. Then swap inductors with another group and show the the phase shift changes appropriately.
6) Determine the inductance of an unknown inductor using your circuit. Document your data and your calculations that lead to your conclusion and discuss the accuracy with which you can make this determination.
Lab "Notebook":
The following information should be clearly presented in your lab "notebook" along with explanatory and insightful commentary.
I) A clear drawing of the circuit constructed including the connections to the function generator and oscilloscope.
II) Show how you calculated/determined the phase shift as a function of inductance for your circuit.
III) Representative sketches of the output signals observed at various points in the process.
IV) Present your measured phase shift(s) that confirm (or not) the known inductance in your circuit?
V) Present your results for the determination of the unknown inductance.
