Mood Brightener: ...more from Stay Homas. (Confination VIII)

Your Conceptual Goals for this section: Electric Potential (ΔV)

Falstad Math/Physics Simulations:

This is the quantitative part of our discussion about Electric Potential

Electrical Potential

From the previous breadcrumb you will remember that

ΔV_AB= W_AB/q

...and the units of the electric potential are J/C which we call Volts.

Electric Potential (implied reference point):

In the case of gravity we didn't pursue this much because the gravitional field everywhere on the earth is very uniform and so the simpler approach of ΔPE_g = mg(h_f-h_o) works pretty well. In electrostatics, the settings we are interested in are more complex so we need to dig a little deeper.

Lets consider a charge in the space near a point charge which creates an E field.

This is a useful image -- we'll label where the charge is now A and the point P as B. Will the force on the charge be constant as it moves from A to B? How do we determine work when the force is not constant?

Remember this?

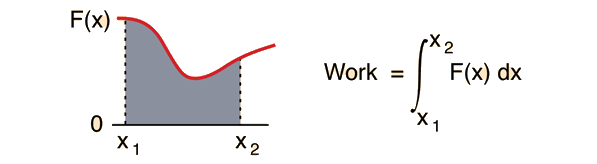
In this example they don't clearly note the dot product inside the integral but in our case what is cos(θ) between the force and the direction of displacement? We could do this by plotting the force as a function of the radial distance and then using manual techniques like we did for the Bungee II lab. On the other hand we're practicing integration so let's go do it.

Here's the place we're going (and we'll see if we get there:))....

ΔV_r = k q_src/r

In this expression there is an implicit assumption that this is a potential difference between a point r some distance away from the source charge and a point infinitely far away. Most texts don't keep the Δ in front of the V but I think it's a helpful reminder that this is actually still a potential difference. There is no such thing as a potential but only differences relative to some reference point.

In the same sense the potential energy due to gravity was always a difference relative to some reference point. There is no absolute value associated with the gravitational potential energy.

Notice also that + charges are like hills and the potential near them is higher than points far away. Source charges that are - are like holes in that the potential near them is lower than points far away. This is a new conceptual feature that doesn't happen with gravity because we don't appear to have both + and - masses.

The Cool Thing!

The cool thing is that potential, like energy, is a scalar since energy is a scalar and so is charge. That means we only have to add and subtract numbers and there is no vector stuff to worry about. This turns out to be very handy and next week we'll explore some important relationships between scalar fields and their associated vector fields. For now just be happy to get a break from the vector stuff.

The (-) and (+) Potentials:

What did (+) and (-) mean when we talked about energy in the past? One suggested energy removed from system and the other energy added. What does this mean in the case of the electric potential. It's more complex than gravity because we have two signs of charge but only only sign of mass (for now). Remember that W_ab = q_field *ΔV_ab. If I take a positive charge that experiences a (+) ΔV then W_ab is positive which means energy is added. If I put a (-) charge through the same calculation then W_ab is negative which means energy is removed from the charge. Same ΔV_ab but different experience because there are two types of charge. It's like a negative mass cannon ball that wants to roll uphill all on it's own. Positive mass cannonballs roll downhill and negatives roll uphill. Weird but true. This is why we DO keep track of signs in our electric potential calculations because it has a different meaning than in the vector land of E Fields and Forces.

Voltage in our houses and lives:

It seems incredibly likely that you have noticed that this unit of electric potential is the same one that we use to describe car batteries, wall outlets, and other stuff of an electrical nature. We will explore this more when we get to circuits. For now it is probably most useful to notice the conceptual connection between voltage differences and electric fields which push or pull on charges. Voltages indicate the existence of source charges and E fields which can then be used to move charges or charged objects around. A perfect example is in an ink jet printer where voltages are used to create fields that direct the charged droplets of ink to the right place on the paper to produce the font that you have selected for your term paper.

We have worked our way from electrostatic forces to E fields to ΔV over the last three weeks. From an engineering perspective we usually do it exactly the opposite way. We design the voltage to produce the force we want, overlooking the mysterious E fields that are produced, to create the effect we want in the world.

Voltages and metal objects:

Consider a solid lump of metal on which I place some excess charge. Where does that charge go? Will there be an E field inside the lump of metal? If there is no E field inside how much work does it take to move a charge from one side of the lump to the other? What does that mean about ΔV_AB between any two points of the metal? Do I change anything about the distibution of the charges if I use an ice cream scoop to take out the inside of the lump once the charges have stabilized? Why does this suggest that you are relatively safe in a thunderstorm if you stay in your car or truck?

If I have a spherical metal sphere which has some excess charge how can I determine what it's voltage is? Perhaps I will get a chance to do this as an integration problem. If not the result is that a spherical distribution of charge can be treated as if it is concentrated at the center of the sphere for all points in space outside of the spherical distribution. This is a very handy result.

Calculation: What is the potential of a 12 cm metal sphere on which 12 nC of excess negative charge has been placed?

Multiple Charges:

So what do we do with multiple charges? Because ΔV_r is a scalar we can just add up the contribution to the potential from each of the charges involved. We do have to keep track of whether the source charge is + or -.

When you solve these problems it is still valuable to make a sketch so you get the geometry right but there is no vector stuff to worry about. + source charges add to the potential at a point and - source charges reduce or subtract from the total electric potential at a point.

Integration:

Just like Coulomb Force and E Field problems in the previous sections EXCEPT that ΔV_AB is a scalar and not a vector!! That's very nice!! Follow the integration frame and remember to be careful about the sign of your source charges. This is a little different than the vector problems we have been doing previously. Practice, practice, practice....

Assignment Breadcrumb Reading: Bb Test

Potential: Axis of Dipole

A dipole whose charges are +1.2 mC and -1.2 mC and are separated by a distance of 62 cm. What is the Electric Potential 62 cm past (further away from the center) either charge.Be sure to know the difference between the point nearest the (+) charge and the point nearest the (-) charge.

Before Next Class:

Assignment HW: Bb Test

Potential Along Line through Dipole:

Find the electric potential everywhere on a line which is parallel to the perpendicular bisector and passes through the positive charge. In the test you will be given the charge, the separation of the dipole and the x,y coordinates of two different points. You will provide a numerical answer for the potential at those two points. Unless you have a closed form solution that you can plug the numbers into you will be toast (I hope). Do your advance work and you should have enough time to crank the numbers through when you get them. The python notebook for practicing this problem is called DipolePotentialLine.ipynb on the github linked in the LMS.

Assignment HW: Bb Test

Reaching the Nucleus:

In order to split a nucleus a proton must penetrate through the E field to reach the nucleus of a Si atom. There will be no E field to contend with until the proton begins to enter the fringes of the atom at a distance of 10 nm. The proton will reach the nucleus 3.6.10^-15 m. from the center of the Si atom. What initial kinetic energy must a proton have to reach a Si nucleus (q=+14e) under these circumstances? Because an atom is a neutral object from a distance you should assume no E field until the proton gets 10 nm from the Si atom. From that point on consider only the field produced by the nucleus which is certainly an oversimplification but acceptable for this problem. An energy bar chart would be very prudent! The python notebook to practice this problem is called SplitAtom.ipynb on the github linked in the LMS.

Assignment HW: Bb Assignment

Disc of Charge (Integration)

Find an expression for the Electric Potential at a point z directly above the center of flat disc of charge. The total charge Q is spread uniformly over the surface of the disc which has radius R. Solve the problem for a ring of charge first and then add up rings to get a disc.

Looking Ahead:

Look ahead to the next Breadcrumb: Equipotentials

Assignment Breadcrumb Reading: Bb Test

Equipotentials:

Choose other data sets that share the same primary feature as equipotentials. There will be more than one correct answer. No, I didn't really mention this in the reading but you might consider looking up isotherms, isobars, and topological map lines.